Muscle Cells

Smooth Muscle Cells

Smooth muscle cells form the walls of hollow organs like blood vessels or the intestines. Like all muscle cells, these cells have characteristic oval, cigar-shaped nuclei that stain light blue. When smooth muscle in the walls of blood vessels contract, they can decrease the diameter of a blood vessel. This increases blood pressure. In people with a condition of high blood pressure, it can be helpful to give medicines that decrease the contractions of smooth muscle and diminish blood pressure. This eases the strain on the heart. In the intestines, contractions of smooth muscle help push food down the length of the intestines. These contractions are regulated by nerve cells found close to the smooth muscle cells.

Skeletal Muscle Cells

Skeletal muscle cells pull on tendons attached to bones. They allow us to walk, use our arms and hands, and adjust our facial expressions. Skeletal muscle cells are truly enormous, cylinder-shaped cells. They can be 0.1 millimeters in width and as much as 10 millimeters long, so they are the Godzillas of the cell world. In order to control so much cytoplasm, each skeletal muscle cell may contain as many as 1000 cell nuclei! Also, many small stripes, or striations, cross the cytoplasm of these cells. How are these special features created?

Skeletal muscle cells are created by the fusion of smaller cells called myoblasts (in the embryo) or satellite cells (in the adult). Numerous special proteins are required to accomplish this cell fusion and turn on the genes needed for muscle cell development.

The stripes in the cytoplasm are light-staining (I bands) or darker-staining (A bands). High magnification pictures show that these bands are composed of masses of highly organized filaments. We now know that these filaments are mainly composed of filaments of actin and filaments of myosin. When provided with energy by mitochondria, these filaments slide against each other. This sliding is what causes the muscle cell to contract. The actin and myosin proteins are forced into a highly regular, almost crystalline, pattern by other proteins that organize them (these proteins are called α-actinin, desmin, titin, and nebulin).

Smooth muscle cells also use actin and myosin to contract, but in these cells, the actin and myosin are less abundant and not so highly organized, so no stripes are visible in these cells.

Cardiac Muscle Cells

Cardiac muscle cells are found in the heart. They are much smaller than skeletal muscle cells, and are shaped like tiny shoeboxes. These cells also contain lots of actin and myosin and show striations similar to those of skeletal muscle cells. Unlike skeletal muscle cells, these cells never rest or take a break. From the moment they are formed until the death of a person, they contract 60 times a minute to cause the heart to beat.

Connective Tissue Cells

Fibroblasts

Of the connective tissue cells, fibroblasts are perhaps the most numerous cells of the body overall. Billions of them inhabit the connective tissue found in all of our organs. A fibroblast has a flattened, dark-staining nucleus and a sparse cytoplasm. They begin their lives in close proximity to each other but gradually are pushed apart by a protein that they produce. This protein, called collagen, forms thick, tough fibers found in the environment of the fibroblasts. Collagen fibers make up most of what we call leather and are very strong. They provide a structural support for softer portions of the body.

Fat Cells

A fat cell is a spherical cell that contains a large cytoplasmic droplet filled with oils, or fat. This fat represents a storage form of nutrients. When other cells in the body run out of nutrients, fat cells release fat molecules into the bloodstream. Muscle cells and nerve cells can then use these molecules as fuel to burn and to power their functions.

Fat cells also produce a hormone called leptin, which is released into the bloodstream as the fat cells get bigger and bigger. Leptin is carried to the brain, where it functions as a signal informing the brain that fat cells are filled with fat molecules. This signifies that there is no shortage of fuel in the body. High levels of leptin suppress feeding behavior, so that excess calories are not consumed.

In some cases, the appetite-suppressing effect of leptin becomes dysfunctional.In genetically abnormal strains of rodents, a defective form of leptin is made by fat cells, or else the brain receptors for leptin don’t work properly. As a result, the brain mistakenly interprets an absence of signaling by leptin to mean that there are no spare calories in fat cells. Consequently, a rat or mouse with these defects will overeat enormously and become very fat. In humans with obesity, there is some speculation that this leptin-signaling system is not working properly. The causes for this are not yet known. Most obese people have normal or even supranormal blood levels of leptin, and leptin receptors seem to be functioning. More research on this topic may shed light upon causes of obesity.

Cartilage Cells

Cartilage cells (chondrocytes) inhabit a stronger, more rigid type of connective tissue called cartilage. These cell begin their lives looking much like fibroblasts but then acquire a spherical, pale-staining cytoplasm and a round nucleus. They secrete a special type of collagen into their environment, along with long chains of sugar molecules. Cartilage cells are found in the cartilage of the ribs and at the ends of bones. During aging, cartilage can become abnormal and may be lost from the ends of bones, causing the pain of arthritis.

Cartilage cells produce a protein called chondromodulin that prevents blood vessels from tunneling into cartilage. This poses a potential problem for cartilage cells: if they are far from blood vessels, how are they to obtain the oxygen and nutrients they need for survival? Fortunately, the sugar molecules around the chondrocytes allow the slow passage of oxygen and nutrients from blood vessels outside of the cartilage.

Bone Cells

Bone cells (osteocytes) live in small cavities in bone, another form of rigid connective tissue. They are surrounded by collagen that has acquired massive deposits of calcium, turning the extracellular environment into kind of a rocky prison for these cells. Fortunately for osteocytes, this rocky material does permit the entry of capillaries into bone, so that osteocytes can receive their vital nutrients from the bloodstream. Even osteocytes far away from a blood vessel can still receive nutrients through a network of tiny channels, termed canaliculi. These canaliculi run through the calcified components of bone to connect osteocytes to each other and to blood vessels. Transfer of nutrients through these canaliculi keeps the osteocytes alive.

Blood

Blood represents a special type of connective tissue. Blood cells are found flowing through blood vessels that are lined with a simple squamous epithelium. Most blood cells are red blood cells. These cells destroy their cell nuclei during development! They become simple containers filled with hemoglobin, an oxygen-binding molecule that helps the red blood cells carry oxygen around the body.

A small proportion of blood cells are white blood cells. These cells often have oddly shaped cell nuclei, and like red blood cells, are born in bone marrow before being released into the bloodstream. White blood cells help the body fight off infections by bacteria and other organisms. These cells can eat and destroy bacteria or else produce chemicals called antibodies that bind to invading foreign molecules.

Introduction to Cells

Basic Structures of Cells

Cells are the basic units of human life. Billions of cells compose your skin, muscles, bones, and brain. A recent estimate shows that the human body is composed of 3.7 trillion cells! Moreover, all of these cells, when closely examined, can be divided into about 200 different cell types, each with their own size, shape, and job to do.

During early development, the cells of the embryo were much simpler looking. They all had a round shape, and they all had 2 features in common: 1) a cell Nucleus in the center of the cell, and 2) a mass of material surrounding the nucleus called the Cytoplasm. The cytoplasm is surrounded by a barrier called the Cell Membrane, and the nucleus is contained within the Nuclear Envelope. When scientists first began studying cells, they had difficulty viewing these features of cells using a microscope. This is because cells are 90% water and are almost transparent! To overcome this problem, scientists devised dyes or stains that stain the cytoplasm pink and the nucleus blue.

Varieties of Cells

All parts of the body contain cells with very different appearances. Tissue in the bone marrow, for example, contains 1) a cell with a huge, folded, pale-staining nucleus and an enormous cytoplasm, 2) a cell with tiny, dark-staining nucleus, 3) a cell with a horseshoe-shaped nucleus, and 4) even a cell with no nucleus at all! In this cell, the contents of the nucleus have spilled into the cytoplasm, because the nuclear envelope has disappeared. These rod-shaped nuclear components are called chromosomes. During cell division, the chromosomes have been duplicated into two identical sets. These two sets of chromosomes are pulled apart from each other and sent to opposite poles of a cell. The cell then divides into two new cells, each with its own nucleus.

This amazing variability in cell shape and function poses a problem. How are we to understand this bewildering variety of cells? Fortunately for us, Mother Nature has provided us with a solution to this problem. All of these types of cells can be sub-divided into only four main categories of cells, each with a major type of job to fulfill.

Amanda Hipps

Could you summarize what you do now?

I’m a master’s student at Florida Atlantic University studying the animals that depend on gopher tortoise burrows, called commensals. Although I am interested in all commensal animals in southeast Florida, I’m gearing much of my effort into looking for a number of insects. Some species of insects feed only on gopher tortoise dung (yeah, they might eat poop, but they’re picky about what kind). These insects are providing dung removal services in the burrows, potentially benefiting tortoises by reducing pest flies and parasite loads. We don’t know much about them including their range and habitat requirements, so I want to determine which species can be found in Southeast Florida and in which habitats. Although this is my master’s thesis project, my advisor, Dr. Jon Moore, has ongoing projects that I assist with. He has been monitoring gopher tortoises at one site for over 17 years and seems to know these animals on a first name basis.

What does a typical field day look like?

Dirty, sweaty, and digging through lots of tortoise poop! I have six field sites along Southeast Florida so I spend a lot of time driving. While at a field site, I use a camera scope to see if any vertebrate commensals, like snakes or frogs, are hiding in the burrow. I’ll sift through the sand, sometimes head first, shoulder-deep inside of a burrow trying to catch insects. I use fresh tortoise dung as bait and have a number of insect traps set out. Most days can be discouraging – I’ll spend hours everyday in the hot Florida sun and usually end the day with nothing exciting to report. When my dad asks for updates, he always says, “Are you even doing anything out there?”. On the occasion when I do find a rare insect, it feels like a pot of gold. They are insects that can be found nowhere else in the world except inside of gopher tortoise burrows. Many of these declining insects haven’t been found in many years and few people get the chance to see them. I feel pretty lucky.

What inspired you to start doing this?

As an undergraduate student at the University of North Florida, I was preparing to apply to vet school and was doing an internship at the Jacksonville Zoo animal hospital. A lot of my time there was spent rehabbing native wildlife and gopher tortoise car strikes seemed to be the most common issue the hospital staff dealt with. This is where I was first introduced to them, but after working with them in a hospital setting, I was interested in getting to know them from an ecological perspective, so I began undergraduate research at my university with Dr. Joe Butler. Having the opportunity to learn about them from a whole new perspective, I was captivated and it sent me down this new career path.

Why do you think it’s important – (or how does it benefit the animals?)

I think that every animal, even the smallest insects, have a role to play in an ecosystem. With increased development, pressure to relocate gopher tortoises in south Florida is especially high. Few studies in southeast Florida have surveyed for commensal animals and potentially this area may house a number of previously undescribed species. It’s important to know what’s here in order to understand the impact they may have on the gopher tortoise.

What is the hardest thing about doing this?

We’re living in a time where there is a large loss in biodiversity. As a native to south Florida, it’s frustrating to see so much habitat loss and development.

What is the most rewarding thing about it?

The most rewarding thing for me is meeting people both in and out of the field that share similar interests. It has connected me with so many people that I wouldn’t have otherwise crossed paths with.

What if others want to help but don’t want to go into the field. How can they help?

The most important thing one can do to help gopher tortoises is to be an informed voter. Support local officials that value natural resources. Saving the species will require cooperation from many people including government officials, private landowners, ranchers, and developers. Conservation of land also includes land management and one important tool for management is fire. Continue learning about the gopher tortoise and use the power of your network to inform and inspire others.

Finally, do you have any advice for a young student wanting to study something like this?

There are many different careers in wildlife conservation, so spend some time exploring what your interests are. Gain hands-on experience via internships or part-time jobs and gear your coursework toward the relevant sciences.

Want to Learn More?

If you’re intrigued by the work Amanda does and the gopher tortoise she follows, I encourage you to learn more about work others are doing via this Untamed Science video made at the Jones Center at Ichauway.

Shark Tracking Science

Blue Skies and Blacktips

It’s a blueberry-sky day in Florida, and a small group is fishing for sharks off the coast. The group is conservation-minded, as are most sport fishermen, so the plan is to release any sharks they catch, often done with full confidence that the animal will be undamaged by the encounter. Or will it? “The assumption that a shark swims away and lives a long, happy life after that isn’t necessarily true.” The person calling the assumption into question is Dr. Nick Whitney of the Anderson Cabot Center for Marine Life. “We’re trying to figure out what happens to sharks after they’re caught and released alive.”

It isn’t long before one of the rods bends and someone hauls in the first catch of the day: a blacktip shark. The blacktip is a popular sport fish in Florida. It is five feet of muscle and often provides fishermen with a show as it leaps out of the water when chasing prey. “Most of the guys who are doing catch-and-release fishing are doing it for the benefit of the animal,” says Whitney. “They want the animal to survive, grow up, be caught again.” Both scientists like Whitney and shark fishermen of today want sharks in the water. However, that hasn’t always been the case.

Does Jaws have stress?

University of Miami biologist Austin Gallagher said in a podcast that sharks are as capable of experiencing stress as any other living creature. “Every organism on this planet experiences stress in some way, from a single-celled organism to a student studying for a test.” Stress is viewed as physiological changes in body chemistry, said Gallagher, and can be induced in sharks by changes in salinity, warming waters, and especially when animals are caught and released.

“You look at a shark and it’s pretty mean looking, with the teeth hanging out, and you would not think that it has any stress. But that’s not the case: shark populations are decreasing worldwide due to fishing.” Gallagher says that concern for sharks has improved a great deal in the past thirty years or so. “In the days when Jaws came out, the feeling was the only good shark is a dead shark,” and fishermen would take sharks purely for the optics of standing beside its bleeding corpse on the dock to pose for a picture. “Fortunately, the days of dragon-slaying are coming to an end.”

A Shark Door Prize

As the blacktip is brought alongside the boat, the crew scrambles to quickly immobilize it. Whitney rolls the shark on its back, inducing a strange state called tonic immobility. The shark, thrashing and pulling just seconds earlier, seemingly falls asleep. Though the group will, like most sport fishermen, release the shark after it’s brought in, the animal will leave with a small souvenir: an acceleration data logger.

The logger is a small device called an accelerometer that will be attached to the dorsal fin of the shark to record the shark’s tail beat, its depth, and pitch (the angle at which it swims). This is the first time acceleration data loggers have been used to study shark post-release mortality.

NOAA says that telemetry devices are used in various forms on everything from salmon smolts to whales and provide vital insights into marine animal behavior. “These observations significantly improve our understanding of ecosystem function and dynamics. Sensors carried by animals deliver high-resolution physical oceanographic data at relatively low cost, and often in difficult areas to study, such as the Arctic.”

In this case, the accelerometer has a strap that is designed to begin deteriorating as soon as it hits the salt water. After being on the shark for about 24 hours, the strap will fall off. The monitor, attached to a small float, will surface and begin to transmit a radio signal that can be detected from about ten miles away.

Recovery and Recording

Karissa Lear of Mote Marine Laboratory is setting up the equipment to retrieve the devices two days after the catching and tagging. Despite the exciting aspects of working with sharks, she says that looks can sometimes be deceiving. “I tell people what I do, and they’re like, ‘Oh you have the greatest job ever!’ They don’t realize that I spend about 90% of my time sitting at my desk writing grants and recording data.”

The crew follows a pinging radio signal toward each of the devices using an antenna that Lear holds near the bow of the boat. Before long, the boat approaches an orange float about eight inches long. Attached to the float is the monitoring device, which the crew picks up using a net. In the end, they’re able to retrieve 100% of the data loggers.

What It All Means

Initial reports from the data loggers show that 91% of the blacktips were able to survive the process of being caught, tagged, and released. It took the sharks roughly eleven hours to recover from the ordeal. Rob Nelson of Untamed Science says that, while the shark data from this trip may be one small piece of a bigger puzzle, the real reward may lie elsewhere: “The biggest result of this study may be simply that using data loggers like this is extremely effective at looking at fishing techniques to determine whether or not a shark survives a particular fishing method.”

Find out more about the topics mentioned here:

Use of Telemetry in Fisheries Management: Juvenile Sandbar Sharks in Delaware Bay

Scientists

Here at Untamed Science we’re excited to create profiles of scientists that do amazing work in their respective fields. We’ve interviewed physicists, paleontologists, botanists, marine scientists and geologists. We’ve dove to the bottom of the ocean with engineers that build submersibles and climbed trees in the rainforest with researchers that track monkeys. More than anything, we want to make sure people see the diversity of research and researchers in the field. This is our portal for linking valuable science and scientists. Have a browse and read the stories of these scientists and science communicators.

Shark Researchers you Should Follow

Nick Whitney – @drnickwhitney

This researcher is unlocking the secret lives of sharks using the same technology found in your smart phones – accelerometers.

Kristine Stump – @drKristineStump

Hailing from the Shedd Aquarium, Kristine studies sharks and other large predators on the reefs.

Jillian Morris-Brake – @biminisharkgirl

Sometimes the most important thing you can do is dedicate yourself to protecting and educating the world about sharks. This is what Jillian is doing with an amazing team down in Bimini.

Jeff Carrier – @SharkDoctor

Jeff is the nurse shark guy! Want to know anything about these “laziest of all sharks,” you know where to start!

John Chisholm – @MA_Sharks

You’ve seen those shows where shark researchers study big great whites and ID them simply by their markings. That’s exactly what John is doing!

Heather Marshall – @HeatherMPhd

Out of Mote Marine Laboratory, Heather is a shark researcher and coordinates the GillsClub, a fantastic youth shark club.

Karissa Lear

This is the real researcher who collected most of Nick’s data for our new video. Karissa also gives us a unique view into the life of a Ph.D candidate – now hailing from Australia.

Yannis Papastamatiou – @Dr_Yannis

Mix in a bit of martial arts and shark research, and you have what every Hollywood producer is looking for in a shark researcher.

Toby Daly-Engel

Toby is a researcher of sharks based in FL. We first met her while she was doing work in Hawaii!

A TwitterVerse of Shark Scientists

There are lots of researchers doing great work on sharks. We just pulled a handful that we found really interesting.

Links to Research Articles

Using Accelerometers to Track Shark Stress

The Basics of Wildfires

Every summer the west seems to burn with hotter and more intense fires. Homes burn and habitat is ravished by huge fires that leave the ecosystem unrecognizable. However, it is important not to think of fire as the enemy here. Fire is only bad if we view it that way. My hope is that after watching these video shorts here, you’ll have a better understanding of the complex nature of fires and how we should be viewing fires. The video below (and this trailer to the video) gives a broad overview of how to best think about fire.

That’s the 100,000 foot view of fire and how it influences both us and the environment. In creating this film, however, we found that there are a few areas that could use a bit more explaining. We made a very short video on each them for those of you that would like to understand the complexity of each aspect a bit more.

The Extended Short Series About Wildland Fire

The following 6 videos are intended to follow up the above videos about wildland fire out west. Each picks apart some of the complexities of fire and goes a bit more indepth on that topic. We hope these serve as a good way to really learn about what’s going on out west with these researchers.

1 – Understanding “Fuels”

If there is one thing that is important to understand, it’s the meaning of fuel and how it plays into our management strategies.

2 – How Drought, Insects and Disease Effects Fire

3 – Understanding Smoke

4 – Why We Can’t Let Fire Just Burn

5 – Misconceptions and Benefits of Wildland Fires

6- Minimizing Risk of Catastrophic Fires

7- How Fire Effects Spotted Owls and Black-backed Woodpeckers

Additional Topics left in this series

How fire effects Spotted Owls

Western Wildfires

On May 1, 2016, a tiny spark was created near Fort McMurray, Alberta, Canada. It was like a lot of other sparks, but this one grew—and fast. Eventually it turned into a raging wildfire that forced tens of thousands of people to evacuate. It destroyed 2,400 homes and occupied parts of two Canadian provinces. What’s more—it’s still burning, more than a year later.

Here is some harrowing footage from the escape (warning: some [rightly justified] language):

https://www.youtube.com/watch?v=ieTQvIdG-Vo

So, it sounds like forest fires are pretty bad, right?

The truth is actually a little more complex than a simple yes or no…

How does fire help Western forests?

Not all ecosystems are created equal. Fire really does harm some ecosystems. But many more Western ecosystems can benefit from fire. Here is a video we made explaining the complexities of western wildland fires:

To understand the complexities of how this all works, let’s consider a baseline forest, free from any intervention from people. Trees live and die, and eventually the understory becomes full of flammable clutter—leaves, dead branches, dried-up plants, etc. The amount of buildup of this flammable material is called the fuel load in the firefighting biz. Every so often a naturally-occurring fire will pass through and clear out the fuels, leaving the larger trees alive and only slightly blackened.

This is really good for most forests, such as lodgepole pines or ponderosa pines. It clears out a clogged understory (have you ever tried walking through a forest full of knee-high, thorny wild roses?), allowing for young trees to get an edge so that they can grow big and tall. This allows the forest to naturally rejuvenate. It’s sort of like a facial scrub that removes dead skin cells to make your skin look healthy and glowing. It’s Nature’s exfoliant.

Many Western animals also benefit from forest fires. Spotted owls, for example, like to take advantage of small burned patches for hunting. They can see a long way after a cleared-out burn! Herbivores also will gobble down the flush of fresh new growth that happens after the fire, full of tasty protein and minerals.

How has fire management in the past evolved to affect us today?

Things worked well this way until one complicating variable came in: people, and lots of them. Many Native American people were responsible for setting fires—either intentionally or unintentionally—but everything began to change when the settlers arrived.

Early European settlers believed fire was the enemy. It seemed to destroy things, especially on the short time scale that the settlers watched this happen. So, why let fires burn if they were so destructive?

This line of thinking continued well into the middle of the 20^th century. The U.S. government even instituted a famous 10 a.m. policy that stated all fires had to be put out by 10 a.m. of the following day after being reported. With more and more people crowding into the West, it seemed to make sense: they didn’t want big wildfires that could potentially get out of control and go on a Fort McMurray-style burn.

What this policy didn’t account for, though, was the fact that fire was natural in these environments. In fact, somewhat counterintuitively, frequent fires helped prevent large, out-of-control fires.

Normally the fuels (leaves, dead branches, etc.) would be whisked away during the frequent smaller burns. Now that those fires were being limited, those fuels had begun piling up like an endless stream of people offloading pallets for a pallet fire.

A single spark could set off a huge wildfire—so large that it would actually migrate into the crowns (tops) of the trees and kill the whole forest. What had started out as a helpful forest facial scrub had turned into a belt sander that took away everything—skin, bones, blood, and all.

How are Western forest fires managed today?

Today, many parts of the West still contain these legacy tinderboxes, just waiting for a spark to light ‘em up like a Christmas tree (pun intended).

But things changed. Fire managers realized they couldn’t go on fighting every last little flame like a never-ending, increasingly difficult game of Whack-A-Mole. So beginning in the second half of the twentieth century, they tried a new tactic: just letting some fires burn.

Over time, biologists started seeing signs of healthy forests again. There was less dead material clogging up the understory, and new trees started to grow. When fires did return a second time around, they were a lot less intense and didn’t have the potential for another conflagration.

Obviously, some fires still needed to be fought. Anything that started getting a little too close for human comfort was targeted. If the fire crossed onto another piece of land with different management styles, it had to be put out. But for the most part, this let-burn policy is now the default option for much of the West.

Why doesn’t the Eastern U.S. adopt this policy as well? I’m glad you asked…

Why is fire management different in the East and West?

Fire management styles in the East and the West are very different, and much of it comes down to two big factors—people and land.

There are way more people in the East than in the West. Don’t believe me? Check out this map of population density in North America. And a huge percentage of land in the West is under the control of a single landowner: the federal government.

Having a lot of people and a lot of landowners in the East makes things complicated. Imagine you’ve got Bob over here who wants big trees to sell to a forestry company. Next door is Jane who wants a cleared patch of land for her horses. The state government at the end of the lane has another patch of land, and they want to dam up a small creek to make a wetland preserve.

There are so many people doing so many things in such close proximity that it’s impossible to let big wildfires run loose. These small patches are much better for an Eastern fire tool—prescribed burns that still clear out the forest understory yet are able to be contained by a group of forest firefighters.

In the West it’s much easier (at least comparatively—it’s still not easy) to let big fires run wild on large swaths of federal land. Some Western wildfires can even get larger than entire states in the Eastern U.S. As long as fire managers keep an eye on the fire and contain it if it threatens humans or strays, fires are allowed to run wild in a way they can’t do in the East.

What are some other challenges in managing Western wildfires?

Even though fires are often allowed to run wild in the West, there are still a lot of challenges in managing them.

The West has a lot more challenging topography than the East. Sure, there are mountains in the Eastern U.S., but the Appalachians just can’t come close to the sheer size of the Rocky Mountains. This varied terrain makes fires move in a different way. They’ll scoot up hills, sometimes miss the downslopes entirely, and travel across the tops of ridges like a wandering hiker. Fire movement and behavior can be more challenging to predict than in the low-lying flatlands of the East.

Drought and insect outbreaks also create a one-two punch to the forest. Climate change is causing droughts that kill many trees, just like your crunchy, dried-out geranium you forgot to water two months ago. A longer season means more time for insects to get busy—both by feasting on trees and with each other, making even more insects. These standing dead trees create a hazard, hearkening back to the old tinderbox days when fuels piled up with explosive potential.

These factors are causing even more wildfires to happen. A recent study demonstrated that climate change has doubled the amount of Western forest fires since 1984.

What about far-Northwestern fires?

Wildfires are adapted to many places in the West, but there are a few places they shouldn’t be happening very frequently—for example, parts of the Arctic tundra.

You’d think that the Arctic tundra would be a difficult place to burn. There are few, if any trees, after all. But, the tundra is literally composed of dead material that has been stacking up—and decaying very slowly, if at all—for millennia, in some cases. It’s sort of like a giant peat bog. (Speaking of which, fun fact: northern European people used to burn peat as fuel for their homes in place of wood.)

Recently, fires have begun to pop up in the Arctic tundra where they haven’t been before, and scientists are only projecting this to increase as the climate heats up more. While this may not immediately affect a whole lot of people when it happens, it has a global consequence.

The tundra serves as a huge carbon reservoir. So large, in fact, that the tundra is estimated to contain half of all the world’s belowground carbon. As long as we have that carbon locked up in the tundra, climate change should proceed at a normal (albeit too-high) pace. But when a tundra fire happens, a ton of carbon is released—literally.

For example, the 2007 Anaktuvuk River fire in northern Alaska released an estimated “2.1 teragrams of C to the atmosphere, an amount similar in magnitude to the annual net C sink for the entire Arctic tundra biome averaged over the last quarter of the twentieth century.” (emphasis added)

We’ll only be able to tell with time (or fancy math projections) what effect this will have on future climate warming.

So…are Western wildfires bad?

In themselves, no. Fires are a necessary part of many Western ecosystems. The problem comes in when people and fires collide, or when fires pop up in places they shouldn’t be.

We can coexist with Western wildfires (for the most part). This is where you come in.

We need more research done on how to improve our current fire management systems and how to better handle new problems that are sure to crop up in the future. We need people to vote for strong political leaders who will support more ecological research and devote more funding to managing wildfires.

More importantly for the short term, we need people to better understand how to deal with wilderness in the West now. We need to teach people how to handle campfires and other flames properly so they don’t set off another Fort McMurray. We need to teach private landowners how to manage their own land so they don’t unwittingly create their own micro-tinderboxes.

We also need to teach people to tolerate wildfires better. Many people still have the “all fires are bad” mentality, or they don’t tolerate some of the wide-ranging effects of forest fires, like smoke or ashes. These people might have good intentions, but they may be placing people in greater danger by pushing for wildfire suppression in cases where it can be beneficial. A few smoky days from a carefully monitored smaller wildfire might just be the price you pay to keep your home safe from a devastating wildfire gone rogue.

If we do these things right, and do them consistently, we’ll all be able to coexist peacefully with Western forests.

Population and Evolutionary Genetics

Evolution can be a tricky thing. In some cases, it’s easy to see it happening. During the Industrial Revolution in Great Britain, for example, white moths disappeared from the population as they were more easily spotted and eaten by birds in the increasingly sooty and blackened environment.

In most cases, though, it’s not so easy to see evolution happening. It can happen slowly over many generations, or in long-lived species. It could also happen within an organism, affecting its metabolic or physiological processes, for example.

How are we supposed to know if something is evolving, then? To do that, we need to look at the genetics of an entire population and something called the Hardy-Weinberg Principle.

You! Out of the gene pool!

A gene pool isn’t really a swimming pool full of genes (as fun as that sounds). Rather, a gene pool is simply all of the genes (and their associated alleles) within an interbreeding population.

An interbreeding population is defined as one which freely interbreeds with each other, such as a group of geese who migrate to the same summer breeding grounds or a group of all the people who can meet each other. As you can imagine, the human breeding pool has become a lot larger due to globalization and the ability to meet almost anyone over the internet.

So, rather than looking at a herd of deer and wondering which ones have a particular trait, you’d instead look at a list of all the alleles for that trait in the herd’s genomes.

The reason we look at gene pools is because we can compare them over time. Let’s say that we were studying a population of arctic wolves. Over time, the percentage of black wolves increases. In this case, the black fur allele is increasing in frequency among the wolves. The population is evolving.

We can’t always easily see what alleles an individual has, though, like we can with black wolves. Maybe all the organisms look normal on the outside, but they have something unique hidden away within each of them. So, rather than rely on something observable, like fur color, evolutionary geneticists rely on numbers.

The Hardy-Weinberg Principle: A Magic Number

After scientists first discovered how genetics work in the late 18th century, they realized they had a problem. They knew how alleles were passed on between individuals from generation to generation, but how could they describe if populations of organisms were evolving?

Godfrey Hardy and Wilhelm Weinberg both came up with the solution in 1908—individually, oddly enough. Today, we call it the Hardy-Weinberg principle, and it measures the genetic makeup of a population at a single point in time.

If you compare the genetic makeup over time or to certain expected numbers, then boom: you can literally see if your population is evolving. In this case, we use numbers to describe what we cannot necessarily see with our eyes.

It works like this:

If there are two alleles in a population—p and q—each allele will have an allele frequency. For example, 30% of the population could have the p allele and 70% could have the q allele. Or, maybe 50% of the population has the p allele and the other 50% has the q allele.

These two alleles can make up three possible genotypes: pp, pq, and qq (the same as the YY, Yy, and yy of Mendel’s pea plants).

Here’s the cool thing: if you know the allele frequency of the two genes, you can also predict the genotype frequency in the population with some neat math:

p2 + 2pq + q2 = 1

This is because each pp individual will have two p alleles (p2), each pq individual has a p and a q allele (2pq), and each qq individual has two q alleles (q2).

This formula calculates the genotype frequencies for a population that is not evolving. If a population actually has these genotype frequencies, we say that the population is in Hardy-Weinburg equilibrium.

It might help to look at an example.

How do you calculate Hardy-Weinberg equilibrium?

Do you remember the Tasmanian devil from the Looney Tunes? Tasmanian devils are real animals. They look like small dogs, and they’re mean. So mean, in fact, that they tend to get bitten a lot around their faces when mating.

Unfortunately, this aggressive behavior has opened the way for a deadly contagious cancer called Devil Facial Tumor Disease (DFTD) to make its way into the population. These animals are already endangered, and the tumor sure isn’t helping much.

Luckily, some of the animals in the population are resistant to the cancer. These animals are more likely to pass on their resistance genes, and so the population as a whole is evolving to become more resistant to DFTD.

But how did scientists find that out? Let’s put on our safari hats and find out.

Let’s pretend we went on a Tasmanian expedition. Once we got there, we collected DNA samples from all of the Tasmanian devils we could find. Then, we brought the samples back to the lab and analyzed them for a gene that makes the devils resistant.

In this example, having a D allele makes devils more susceptible to the disease, while the d allele makes them more resistant. Let’s pretend this is how many animals of each genotype we measure:

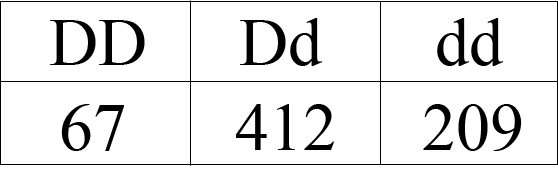
Now, we need to figure out the allele frequencies for the two alleles so that we can plug them into the Hardy-Weinberg equation to see if the population is evolving.

To start, we need to know how many animals are in our “population.” For this, we simply add up all of the animals we sampled of each genotype:

Total number of animals sampled: 67 + 412 + 209 = 688

Each animal has two alleles, so we simply multiply the number of animals by two to find out how many total alleles we’re working with:

Total number of alleles: 688 • 2 = 1,376

Now we need to figure out how many of each of the two alleles are in the population. Each DD devil has two D alleles, while each Dd devil has one D allele, so we simply add these together. Then, we do the same thing for the d allele.

Number of D alleles: 67 + 67 + 412 = 546

Number of d alleles: 412 + 209 + 209 = 830

Finally, we are able to calculate the allele frequencies because we know how many of each allele we’re working with, and how many total alleles there are. So, to find the D allele frequency, we divide the number of D alleles by the total number of alleles. Then, we do the same thing for the d allele.

D allele frequency: 546 / 1,376 = 0.40

d allele frequency: 830 / 1,376 = 0.60

Now we’re able to actually use the Hardy-Weinberg equation. We know the allele frequencies for the two alleles, so we can calculate how many animals in the population there should be for each genotype if it is in Hardy-Weinberg equilibrium (i.e., is not evolving).

To do this, we multiply the total number of animals in our sample by the expected genotype frequencies as predicted by the Hardy-Weinberg equation:

Expected number of DD devils: 688 • 0.402 = 110

Expected number of Dd devils: 688 • 2 • 0.40 • 0.60 = 330

Expected number of dd devils: 688 • 0.602 = 248

Now, let’s compare the observed number of devils we actually measured of each genotype with the Hardy-Weinberg expected values:

As you can see, the expected values are way off from the actual observed values. Scientists can actually see if these results are real or just random numbers from chance by using a statistical test called a χ2 (a “chi-square”).

In this case, though, it’s pretty obvious: the observed numbers are very different from the predicted numbers.

It’s pretty common for the expected number of animals of each genotype under Hardy-Weinberg equilibrium to be very different from the real numbers we measured. This means that the population is currently undergoing evolution. Hopefully they’ll be able to do it fast enough to avoid extinction by DFTD!

Why use the Hardy-Weinberg equation?

The reason scientists use the Hardy-Weinberg equation is because it provides a null model of evolution. This means that it’s a baseline ruler that scientists can use.

If the actual number of animals of each genotype in the example you saw above matched what would be expected, then we would say that the devils are in Hardy-Weinberg equilibrium, and that the population is not undergoing evolution.

What’s more interesting is when a population is not in Hardy-Weinberg equilibrium. This means that something is causing the population to evolve in some way. Then the fun begins—you need to use your critical thinking skills to find out why the population is evolving. This is what evolutionary and population geneticists as a whole spend their time doing.

What are the rules for Hardy-Weinberg equilibrium?

What if a population is not evolving? In that case, it must be breaking one of the fundamental rules that all populations in Hardy-Weinberg equilibrium must follow. But don’t worry! You can use these rules as your clues. One or more of these rules is being broken, and that’s causing the population to evolve: your job is to figure out which ones. Here are some of them:

Only Two Alleles Per Gene

In this case, we’re looking at very simplified versions of genes with only two possible alleles. But, there can be many alleles! If this is the case, don’t fret; there still are calculations out there to take into account three or more alleles, but the math gets complicated very quickly. It’s best to find one of your math buddies or use a computer program if this is the case.

Sexually-Reproducing Organisms

The Hardy-Weinberg principle relies on the genes being reshuffled every generation. This is what sexually-reproducing organisms do. But, not all organisms reproduce like this; some, like bacteria, simply split in half, essentially creating two clones. The Hardy-Weinberg principle does not apply to these critters because the alleles aren’t being reshuffled in every generation.

No Migration

Hardy-Weinberg applies to one interbreeding population. If the population expands from one generation to the next—say, if some wayward migrants get blown in by a storm—it resets the allele frequency in the population and you need to start from scratch.

Large Population

For these calculations to work, you need to be looking at a large population. If you’re working with a small population, it’s likely that rare alleles might occur in just one or a few individuals, if at all. If some random event happens, like a storm or two lovebirds just never meeting up, these animals (and their rare alleles) could all easily be wiped out. This could very easily have happened with the first few Tasmanian devils who were resistant to DFTD. On the other hand, if the population is large, it’s harder for all of those critters with rare alleles to kick the bucket at once.

In small populations it’s far more likely that alleles will drift away from Hardy-Weinberg equilibrium by chance events alone. Population geneticists call this phenomenon genetic drift.

Random Mating

Hardy-Weinberg relies on random reshuffling of alleles. If those alleles gravitate towards each other—say, if purple-haired individuals tend to stick to their own kind and pink-haired individuals do the same—then the alleles won’t be randomly remixing.

No Selection

Perhaps the biggest reason that populations break from Hardy-Weinberg equilibrium is that some individuals are being selected to pass their alleles on, while others are not. In our Tasmanian devil example, individual devils who are resistant to the disease are far more likely to pass their genes on than susceptible individuals—because those ones will be the first to die.

Selection can either be natural (as in the Tasmanian devil case) or artificial (as in selective breeding by humans). Artificial selection is responsible for a range of crops that we eat and the animals that we have as pets. We started out with wild plants and animals and bred the ones we liked best until we arrive at present-day vegetables and pets.

Why study population and evolutionary genetics?

It’s important to understand how population and evolutionary genetics work so that we can maintain genetic diversity. The more alleles we have in a population for each species, the more likely it is to persist on the landscape.

It’s like having a whole bag of tools at your disposal. If some unexpected disease or other challenge pops up, populations with a high genetic diversity (i.e., high number of different alleles) are more likely to persist because it’s likely that some individual within the population will have the alleles necessary to survive, like the resistant Tasmanian devils. They have the right tool for the job, just like a good Swiss Army knife.

It’s possible that the resistant allele was only present in a handful of devils to begin with; if those had been wiped out by chance, then the entire species would have been doomed because that life-saving treasure would have been lost.

By studying the alleles within populations, scientists can work to ensure that ecosystems function naturally and effectively, no matter what challenges are biting them in the face.

White-nose Syndrome in Bats

While most people are sleeping, large numbers of bats are busy feeding on insects in North America. Unfortunately, some of these populations of bats are facing large die-offs due to the spread of a disease known as white-nose syndrome.

The Disease

White-nose syndrome (WNS) was first observed in New York in 2006 and has killed over 5.7 million bats in North America in the last 10 years. It has spread quickly and is confirmed in 29 states and 5 Canadian provinces. It’s believed WNS was accidentally introduced to the US from Europe by humans.

It is named white-nose syndrome because the fungus that causes it (Pseudogymnoascus destructans) often appears in white patches on the noses and wings of infected bats. WNS causes infected bats to be active when they would normally be hibernating, resulting in a loss of their winter fat reserves. Without these fat reserves, the bats are unable to survive the winter. Bats dead from the disease have been found in large numbers in caves. Often less than 10% of the bats in an infected cave survive.

How It Spreads

The spores from the fungus are easily spread by animals and humans. If a bat travels from an infected cave to a new cave, it is likely bringing spores with it. Researchers and explorers who enter an infected cave have to take great care in cleaning all of their clothing and equipment to prevent spreading the fungus themselves.

Species Affected

Seven bat species have been diagnosed with WNS:

Little brown bat (Myotis lucifugus)
Tri-colored bat (Perimyotis subflavus)
Northern long-eared bat (Myotis septentrionalis)
Big brown bat (Eptesicuc fuscus)
Gray bat (Myotis grisescens)
Indiana bat (Myotis sodalis)
Eastern small-footed bat (Myotis leibii)

The little brown bat was once the most common bat in the northeastern US, but very few now remain in that region due to WNS. Two other species, gray bats and Indiana bats, are endangered also, putting their populations at a high risk of extinction.

Resistant Species

Five North American species have been found with the fungus on them that causes WNS but show no symptoms of the disease. It’s possible that these species are resistant to the disease. They include:

Eastern red bat (Lasiurus borealis)
Sountheastern bat (Myotis austroriparius)
Silver-haired bat (Lasionycteris noctivagans)
Rafinesque’s big-eared bat (Corynorhinus rafinesquii)
Virginia big-eared bat (Corynorhinus townsendii virginianus)

Bats in Europe, where the fungus is believed to have been present for a long time, are apparently immune to the disease.

Research Controversy

There is some disagreement in the research community about the best way to continue monitoring the bats threatened by WNS. Some researchers continue to study bats during their winter hibernation in order to test the bats for the presence of the fungus and observe and symptoms of the disease. Every time a bat is disturbed during hibernation, it burns off some of its winter fat stores, putting it at greater risk of not having enough fat to survive the winter.

Many researchers agree that the focus should shift to studying populations that have survived WNS. These survivors may have something in common with the immune European populations.

The Future

Protecting the survivors of WNS and minimizing disturbances to these populations during winter is the best option we have to help these bats survive and hopefully return the affected species to pre-WNS numbers.